Drug Abuse

A Theory of Rational Addiction
Gary S. Becker and Kevin M. Murphy
University of Chicago
Journal of Political Economy, 1988 vol 96 no. 4

We had helpful assistance from Michael Gibbs and useful comments from Michael Grossman, Laurence lannaccone, Sherwin Rosen, Josh Scheinkman, Andrei Shleifer, two referees, an editor, and participants at seminars at many institutions. We have received financial support from the Lynde and Harry Bradley Foundation, through the Center for the Study of the Economy and the State at the University of Chicago.

We develop a theory of rational addiction in which rationality means a consistent plan to maximize utility over time. Strong addiction to a good requires a big effect of past consumption of the good on current consumption. Such powerful complementarities cause some steady states to be unstable. They are an important part of our analysis because even small deviations from the consumption at an unstable steady state can lead to large cumulative rises over time in addictive consumption or to rapid falls in consumption to abstention. Our theory also implies that "cold turkey" is used to end strong addictions, that addicts often go on binges, that addicts respond more to permanent than to temporary changes, in prices of addictive goods, and that anxiety and tensions can precipitate an addiction.

Use cloth breed a habit. [WILLIAM SHAKESPEARE, The Two Gentlemen of Verona]

I. Introduction

Rational consumers maximize utility from stable preferences as they try to anticipate the future consequences of their choices. Addictions would seem to be the antithesis of rational behavior. Does an alcoholic or heroin user maximize or weigh the future? Surely his preferences shift rapidly over time as his mood changes? Yet, as the title of our paper indicates, we claim that addictions, even strong ones, are usually rational in the sense of involving forward-looking maximization with stable preferences. Our claim is even stronger: a rational framework permits new insights into addictive behavior.

People get addicted not only to alcohol, cocaine, and cigarettes but also to work, eating, music, television, their standard of living, other people, religion, and many other activities. Therefore, much behavior would be excluded from the rational choice framework if addictions have to be explained in another way. Fortunately, a separate theory is not necessary since rational choice theory can explain a wide variety of addictive behavior.

Sections II and III develop our model of rational addiction. They set out first-order conditions for utility maximization and consider dynamic aspects of addictive consumption. They derive conditions that determine whether steady-state consumption levels are unstable or stable. Unstable steady states are crucial to the understanding of rational addiction.

Sections IV and V consider in detail the variables highlighted by the previous sections that determine whether a person becomes addicted to a particular good. These sections also derive the effects on the long-run demand for addictive goods of permanent changes in income and in the current and future cost of addictive goods.

Section VI shows that consumption of addictive goods responds less to temporary changes in prices than to permanent changes. In addition, the effects on future consumption of changes in current prices become weaker over time when steady-state consumption is stable, but they get stronger when the steady state is unstable. This section also shows how divorce, unemployment, and similar tension-raising events affect the demand for addictive goods.

Section VII indicates why strong rational addictions must terminate abruptly, that is, must require going "cold turkey." Rational binges are also considered.

Our analysis builds on the model of rational addiction introduced by Stigler and Becker (1977) and developed much further by lannaccone (1984, 1986). He also relates the analysis of' addiction to the literature on habit persistence, especially to the work by Pollak (1970, 1976), Ryder and Heal (1973), Boyer (1978, 1983), and Spinnewyn (1981). We appear to be the first to stress the importance for addictions of unstable steady-state consumption levels, to derive explicit long- and short-run demand functions for addictive goods, to show why addictions lead to abrupt withdrawals and binges, and to relate even temporary stressful events to permanent addictions.

II.   The Model

Utility of an individual at any moment depends on the consumption of two goods, c and y. These goods are distinguished by assuming that current utility also depends on a measure of past consumption of c but not of y, as in

For most of the discussion we assume that u is a strongly concave function of y, c, and S. Past consumption of c affects current utility through a process of "learning by doing," as summarized by the stock of "consumption capital" (S). Although more general formulations can be readily handled, a simple investment function is adopted for the present:

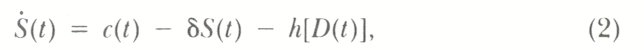
where Ś is the rate of change over time in S, c is gross investment in "learning," the instantaneous depreciation rate δ measures the exogenous rate of disappearance of the physical and mental effects of' past consumption of c, and D(t) represents expenditures on endogenous depreciation or appreciation.

With a length of life equal to T and a constant rate of time preference, 0 the utility function would be

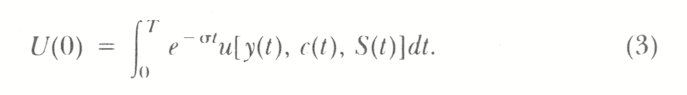
Utility is separable over time in y, c, and S but not in y and c alone because their marginal utilities depend on past values of c, as measured by S.

A rational person maximizes utility subject to a constraint on his expenditures. If A0 is the initial value of assets, if the rate of interest (r) is constant over time, if earnings at time t are a concave function of the stock of consumption capital at t, w(S), and if capital markets are perfect, then the budget equation would be

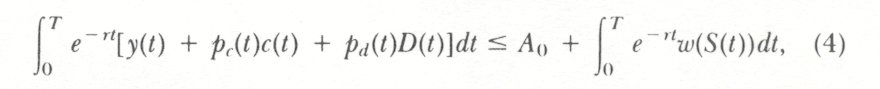
where the numeraire (y) has a constant price over time. A person maximizes his utility in equation (3) subject to this budget constraint and to the investment equation (2). The value (in utility terms) of the optimal solution, V(Ao, So, w, p), gives the maximum obtainable utility from initial assets Ao, initial stock of capital So, the earnings function w(S), and a price structure p(t). Since u(-) and w(S) are concave functions, V(Ao, So, p) is concave in Ao and So. If μ = δV/δAo, then by concavity dμ/dAo < 0.

The optimal paths of y(t) and c(t) are determined by the first-order conditions. If we let

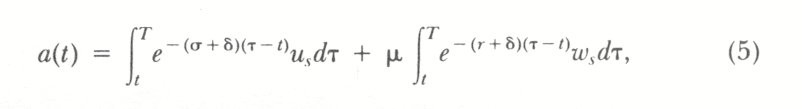
then

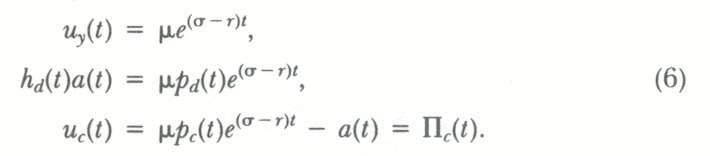
The expression a(t) represents the discounted utility and monetary cost or benefit of additional consumption of c through the effect on future stocks. It measures the shadow price of an additional unit of stock. A rational person recognizes that consumption of a harmful good (u s, w s 0) has positive effects on future utility and earnings. The shadow, or full, price of c(t), IIc(t), equals the sum of its market price and the money value of the future cost or benefit of consumption (see also Stigler and Becker 1977, eq. [8]). The stock component of the full price is itself endogenously determined by the optimal path, and yet it can also be said to help determine the optimal path by affecting the cost of c.

Clearly, if future consumption is held fixed, the absolute value of a(t) is smaller when the depreciation rate on past consumption (8) and the rate of preference for the present (a) are greater. This suggests that consumption of a harmful c is larger, and consumption of a beneficial c is smaller, when 8 and v are greater. We will see that S and a are also important in determining whether c is addictive.

It is clear from the second first-order condition that the optimal expenditure on endogenous depreciation (D) to reduce the stock of capital is larger, or the optimal expenditure on endogenous appreciation to increase the stock is smaller, when the marginal value of the stock, a(t), is smaller. This value falls as the stock increases since the value function is concave in S. Therefore, individuals will take steps to depreciate the stock more rapidly when it is larger.

III. Dynamics

The first-order conditions (5) determine the initial consumption level of c, co, as a function of the initial stock of consumption capital, So, prices p(t), and the marginal utility of wealth μ. To simplify the discussion of dynamics, we first assume an infinite life (T = ώ), a rate of time preference equal to the rate of interest (o = r), and no endogenous depreciation (D(t) = 0). Since μ remains constant over time, the relations between co and So for given μ and p also give the relation over time between c and S for these values of μ and p.

To analyze the dynamic behavior of c and S near a steady state, we can either take linear approximations to the first-order conditions or assume quadratic utility and earnings functions that have linear first order conditions. (Related dynamics were developed by Ryder and Heal [1973] and Boyer [1983].) If the utility function u is quadratic in c, y, and S, if earnings are quadratic in S, and if p,(t) = p c for all t, then the value function is also quadratic. By optimizing y out with its first order condition, we obtain a function that is quadratic only in c(t) and S(t):

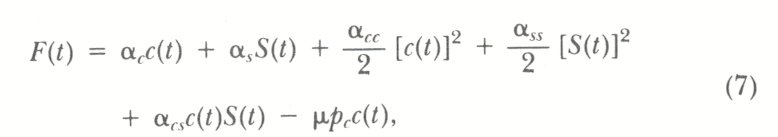
where the coefficients αs and αss depend on the coefficients of both the utility and earnings functions. We know that αss < 0 and αcc < 0 by concavity of the u and w functions. Then the optimization problem involves only c(t) and S(t):

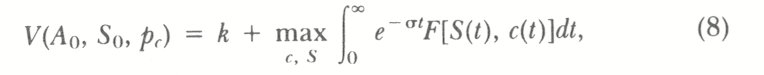
where k is a constant that depends on A0, μ, σ and the coefficients for y in the quadratic utility function. The maximization occurs subject to equation (2) with h = 0 and to the transversality condition

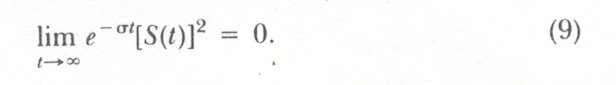
Equation (8) is a straightforward maximization problem in the calculus of variations, where F is a function only of S and Ŝ through the linear relation between c, S, and Ŝ in equation (2). The Euler equation can be expressed as

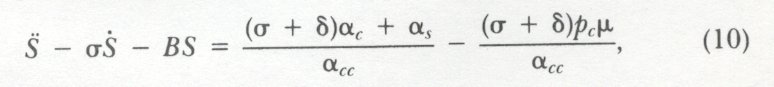
with

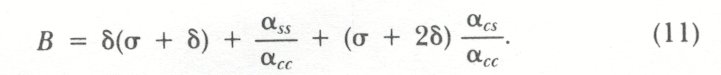
This is a second-order linear differential equation in S(t), with two roots given by

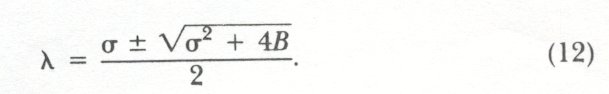
The term under the radical is positive because essentially it is a quadratic form in σ + 2δ and 2:

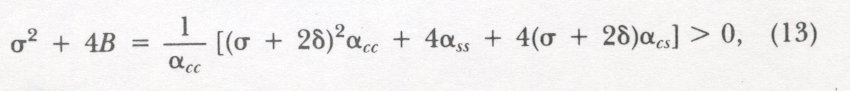
and the Hessian of the concave function F is negative definite. Hence both roots of (12) are real. Moreover, the larger root exceeds Q/2 and can be ignored with an infinite horizon; otherwise, [c(t)] 2 would eventually grow at a faster rate than 0 which would violate the transversality condition in equation (8).

The optimal path of the capital stock is determined from the initial condition and the smaller root alone:

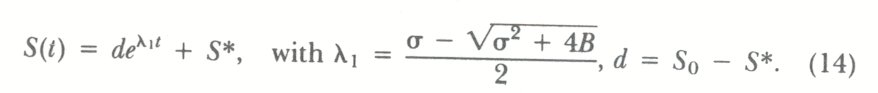
If the steady state, S*, is stable, S grows over time to S* if S o S*. Equation (14) shows that S* is stable if and only if B > 0 because then k, < 0.

Equation (14) also implies that

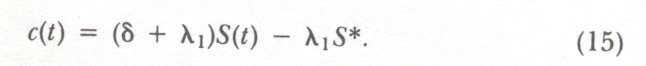
The slope between c and S increases as λ1 increases, and it reaches a maximum value when λ1  = σ/2, that is, when σ² + 4B = 0. Given the definition of λ1 in equation (14) and of B in equation (11), equation (15) implies that c and S are positively related (λ1 > -δ), negatively related (λ1 < - δ), or unrelated (λ1 = - δ) as

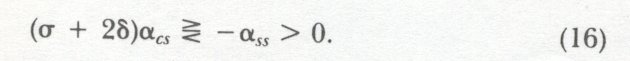
Since "unrelated" means that past consumption of c has no effect on its present consumption, behavior would then be the same as when preferences are additively separable over time in c and y, even though the utility function is nonseparable in S and c. Whether behavior is effectively separable over time depends not only on the currentperiod utility and earnings functions but also on time preference and the rate of depreciation of past consumption.

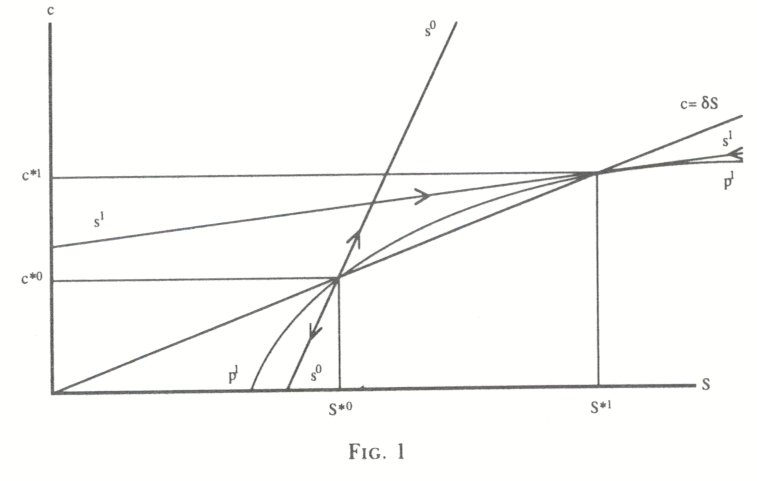
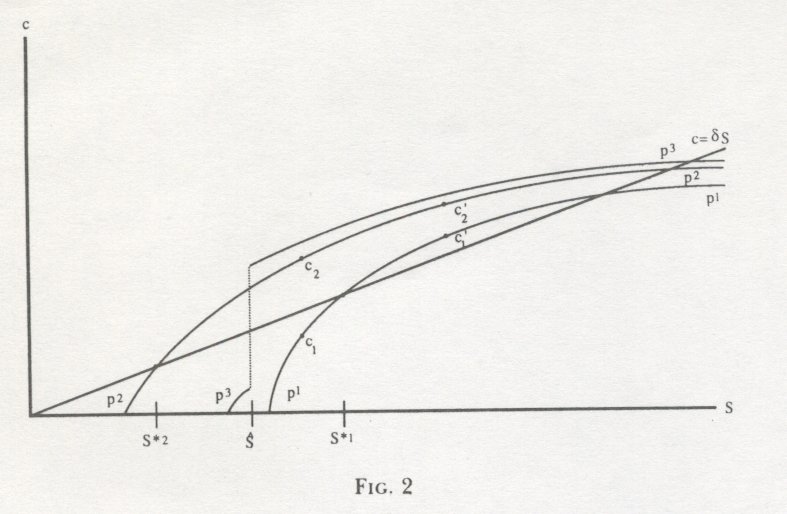
The line s¹s¹ in figure 1 has a stable steady state at δS*¹ = c*¹, whereas the line s°s° has an unstable steady state at δS*° = c*°. The arrows indicate that deviations from S*¹ cause a return to S*1 along the linear path s¹s¹. Deviations from S*°. cause further deviations in the same direction along the linear path s°s°.

IV. Adjacent Complementarity and Addiction

If the marginal utility of c in the F function is greater when the stock of consumption capital (S) is greater (αcs > 0), the marginal utility of c would rise over time if S rose over time. Consumption of c, however, might still fall over time because the full price of c (II, in eq. [6]) also rises over time since αss< 0. The rise in full price would be larger when the function F is more concave in S (αss is larger in absolute value for a given value of αcs), when the future is less heavily discounted (α is smaller), and when depreciation of past consumption (δ) is less rapid. The increase over time in the marginal utility of c would exceed the increase in full price if and only if the left-hand side of equation (16) exceeds the right-hand side. There is said to be "adjacent complementarity" when this inequality holds (the concepts of adjacent and distant complementarity were introduced by Ryder and Heal [1973]).

The basic definition of addiction at the foundation of our analysis is that a person is potentially addicted to c if an increase in his current consumption of c increases his future consumption of c. This occurs if and only if his behavior displays adjacent complementarity. This definition has the plausible implication that someone is addicted to a good only when past consumption of the good raises the marginal utility of present consumption ((αcs > 0). However, such an effect on the marginal utility is necessary but is by no means sufficient even for potential addiction since potential addiction also depends on the other variables in equation (16).

The relation between addiction and adjacent complementarity was first recognized by Boyer (1983) and lannaccone (1986). Boyer considers discrete time and the special case in which (in our notation) S¹ = ct - 1. The distinction between adjacent complementarity and the effect of S on the marginal utility of c is not interesting analytically in that case because the sign of αcs is then the sole determinant of whether past and present consumption are complements or substitutes.

Experimental and other studies of harmful addictions have usually found reinforcement and tolerance (Donegan et al. 1983). Reinforcement means that greater current consumption of a good raises its future consumption. Reinforcement is closely related to the concept of adjacent complementarity. Tolerance means that given levels of consumption are less satisfying when past consumption has been greater. Rational harmful addictions (but not beneficial addictions) do imply a form of tolerance because higher past consumption of harmful goods lowers the present utility from the same consumption level.

According to our definition of addiction, a good may be addictive to some persons but not to others, and a person may be addicted to some goods but not to other goods. Addictions involve an interaction between persons and goods. For example, liquor, jogging, cigarettes, gambling, and religion are addictive to some people but not to others. The importance of the individual is clearest in: the role of time preference in determining whether there is adjacent complementarity. Our analysis implies the common view that present-oriented individuals are potentially more addicted to harmful goods than futureoriented individuals. The reason for this is that an increase in past consumption leads to a smaller rise in full price when the future is more heavily discounted.

The rate of depreciation of past consumption (S), complementarity between present and past consumption (a,.,), and the effect of changes in the stock of consumption capital on earnings depend on the individual as well as on the good. For example, drunkenness is much more harmful to productivity in some jobs than in other jobs.

Whether a potentially addictive person does become addicted depends on his initial stock of consumption capital and the location of his demand curve. For example, the curves that relate c and S in figure 1 display adjacent complementarity, yet the person with these relations would ultimately abstain from consuming c if S o < S* o and s °s ° is relevant. We postpone until Section VI a discussion of the determination of the initial stock of consumption capital and the location of demand functions.

The smaller root (λl) in (12) is larger in algebraic value when the degree of adjacent complementarity increases because of increases in σ, δ, or αcs. This root along with the larger root would be positive if adjacent complementarity is sufficiently strong to make B < 0. The steady state is then unstable: consumption grows over time if initial consumption exceeds the steady-state level, and it falls to zero if initial consumption is below that level.

Unstable steady states are not an analytical nuisance to be eliminated by appropriate assumptions, for they are crucial to the understanding of rational addictive behavior. The reason is that an increase in the degree of potential addiction (i.e., an increase in the degree of adjacent complementarity) raises the likelihood that the steady state is unstable. Moreover, there must be adjacent complementarity in the vicinity of an unstable steady state because the curve that relates c and S must cut the positively sloped steady-state line from below at unstable points; see point (c*°, S*°) in figure 1. Unstable steady states are needed to explain rational "pathological" addictions, in which a person's consumption of a good continues to increase over time even though he fully anticipates the future and his rate of time preference is no smaller than the rate of interest. However, they are also important in explaining "normal" addictions that may involve rapid increases in consumption only for a while.

Unstable steady states also lead to another key feature of addictions: multiple steady states. Quadratic utility and earnings functions cannot explain multiple steady states because they imply the linear relation between c and S in equation (16). However, if a quadratic function were only a local approximation to the true function near a steady state and if the true function, say, had a cubic term in S s with a negative coefficient added to a quadratic function, the first-order conditions in equation (6) would then generally imply two interior steady states, one stable and one unstable. The negative coefficient for S³ means that the degree of adjacent complementarity declines as S increases (see curve p¹ p¹ in fig. 1) so that the level of c is smaller at the unstable steady state (c* °, S* °) than at the stable steady state (c*¹, S*¹).

With two steady states, relatively few persons consistently consume small quantities of addictive goods. Consumption diverges from the unstable state toward zero or toward the sizable steady-state level. Therefore, goods that are highly addictive to most people tend to have a bimodal distribution of consumption, with one mode located near abstention. Cigarettes and cocaine consumption are good examples of such bimodality. The distribution of alcohol consumption is more continuous presumably because alcoholic beverages are not addictive for many people.

This paper relies on a weak concept of rationality that does not rule out strong discounts of future events. The consumers in our model become more and more myopic as time preference for the present (v) gets larger. The definition of a(t) in equation (5) shows that the present value of the cost of an increase in the current consumption goes to zero as α goes to infinity (if the interest rate equals α). It is then "rational" to ignore the future effects of a change in current consumption.

The definition of adjacent complementarity in equation (16) makes clear that time preference for the present is not necessary for addiction. However, fully myopic consumers (α = ∞) do have the potential to become addicted whenever an increase in past consumption raises the marginal utility of current consumption (αcs > 0). Although fully myopic behavior is formally consistent with our definition of rational behavior, should someone who entirely or largely neglects future consequences of his actions be called rational? Some economists and philosophers even suggest that rationality excludes all time preference.

Fortunately, we can reinterpret v so that it may be positive even when individuals have neutral time preferences. If lives are finite, the inverse of the number of years of life remaining is an approximation to the rate of "time preference" for people who do not discount the future. Then old people are rationally "myopic" because they have few years of life remaining. Other things the same, therefore, older persons are less concerned about the future consequences of current consumption, and hence they are more likely to become addicted. Of course, other things are not usually the same: older people are less healthy and subject to different life cycle events than younger people. Moreover, people who manage to become old are less likely to be strongly addicted to harmful goods.

To simplify the discussion, most of the paper assumes that v = r, but the analysis also has novel implications about the consequences of changes in v relative to r. When utility functions are separable over time, an increase in preference for the present compared with the interest rate raises current consumption and reduces future consumption. This intuitive conclusion may not apply with addictive goods because the full cost of an addictive good depends on the degree of time preference. Indeed, if the degree of addiction is sufficiently strong, a higher v is likely to raise the growth over time in consumption of the addictive good (see the fuller discussion in Becker and Murphy [1986, sec. 8]). This steepening of the consumption profile over time as time preference increases is contrary to the intuition built up from prolonged consideration of separable utility functions, but it is not contrary to any significant empirical evidence.

We follow Stigler and Becker (1977) in distinguishing harmful from beneficial addictions by whether consumption capital has negative or positive effects on utility and earnings. Since the definitions of adjacent complementarity and addiction do not depend on first derivatives of the utility and earnings functions, they apply to both harmful and beneficial addictions. For example, increases in σ and δ raise the degree of adjacent complementarity, and hence they raise the extent of potential addiction to both beneficial and harmful goods.

The stock component of full price-the term a(t) in equation (5)does depend on the signs of u s and w s: a future cost is added to the current market price of harmful addictive goods, whereas a future benefit is subtracted from the current price of beneficial goods. Therefore, an increase in the rate of preference for the present and in the depreciation rate on consumption capital raises the demand for harmful goods but lowers the demand for beneficial goods. As a result, drug addicts and alcoholics tend to be present-oriented, while religious individuals and joggers tend to be future-oriented.

V.   Permanent Changes in Price

A permanent decline in the price of c, p,, that is compensated to maintain the marginal utility of wealth (u) constant would raise c(t) because the value function is concave. Moreover,

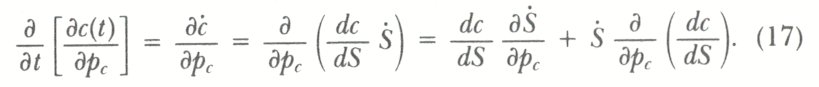
The second term on the far right-hand side is zero in the vicinity of a steady state because S equals zero at the steady state. The sign of the first term is the opposite of the sign of dc/dS because p, has a negative effect on c(t) and hence on Ś. By definition, the sign of dc/dS is positive with adjacent complementarity, zero with independence, and negative with adjacent substitution.

Therefore, the effect of a compensated change in p, on c grows over time when present and past consumption are adjacent complements; that is, the effect grows over time for addictive goods. A permanent change in the price of an addictive good may have only a small initial effect on demand, but the effect grows over time until a new steady state is reached (assuming that consumption eventually approaches a stable state).

Indeed, if the utility function is quadratic, the long-run effect on consumption of a permanent change in price tends to be larger for addictive goods. To show this, differentiate the first-order conditions in equations (6) with a quadratic utility function to get the change in consumption between stable steady states:

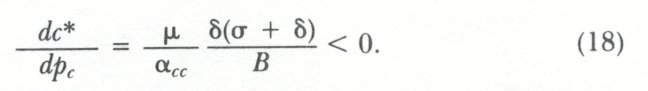
The denominator is negative near stable steady states because αcc 0 (see eq. [14]). Since greater addiction lowers B, greater addiction raises the long-run effect on consumption of a change in own price.

Long-run elasticities would be proportional to the slopes in equation (18) if initial steady-state consumption were independent of B. Changes in B need not affect the initial steady-state consumption because steady-state consumption is determined by first derivatives of the utility and wage functions that do not affect B.

The full effect of a finite change in price on the aggregate consumption of addictive goods could be much greater than the effect in equation (18) because of unstable steady states. In figure 2, all households with initial consumption capital between S*2 and S*1 would be to the left of the unstable state when pc = p¹ and the relevant curve is p¹p¹, but they would be to the right of the unstable state when pc = p² and the relevant curve is p² p² . Hence a reduction in price from p¹ to p² greatly raises the long-run demand for c by these households.

Smoking and drinking are the only harmful addictions that have been extensively studied empirically. Mullahy (1985, chap. 2) reviews many estimates of the demand for cigarettes and shows that they are mainly distributed between .4 and .5. Estimates that implement our model of addiction imply long-run price elasticities for cigarettes of about .6 (see Becker, Grossman, and Murphy 1987). This is not small compared to elasticities estimated for other goods. Price elasticities for alcoholic beverages appear to be higher, especially for liquor (see the studies reviewed in Cook and Tauchen [1982]).

The aggregate demand for drinking and smoking could be quite responsive to price, and yet the most addicted might have modest responses. Fortunately, Cook and Tauchen consider the effect of the cost of liquor on heavy drinking as well as on the aggregate amount of drinking. They measure heavy drinking by the death rate from cirrhosis of the liver (heavy drinking is a major cause of death from this disease). They conclude that even small changes in state excise taxes on liquor have a large effect on death rates from this disease. This suggests either that heavy drinkers greatly reduce their consumption when liquor becomes more expensive or that the number of individuals who become heavy drinkers is sensitive to the price of other harmfully addictive goods are often illegal; beneficially addictive goods, such as particular religions or types of music, are also sometimes banned. Banned goods become more expensive when the ban is supported by punishments to consumers and producers. Our analysis implies that the long-run demand for illegal heroin and other illegal addictive drugs tends to be much reduced by severe punishments that greatly raise their cost. However, the demand for banned addictive goods may not respond much to a temporary rise in price due to a temporary burst of active law enforcement or during the first year after a permanent ban is imposed.

The full price of addictive goods to rational consumers includes the money value of changes in future utility and earnings induced by changes in current consumption. The information that began to become available in the late 1950s on the relation between smoking and health provides an excellent experiment on whether persons addicted to smoking consider delayed harmful consequences or whether, instead, they are myopic. Ippolito, Murphy, and Sant (1979) estimate that 11 years after the first Surgeon General's report on smoking in 1964, per capita consumption of cigarettes and of tar and nicotine had been reduced by 34 percent and 45 percent, respectively. This evidence blatantly contradicts the view that the majority of smokers were myopic and would not respond to information about future consequences because they discounted the future heavily.

Of course, persons who continued to smoke, and those who began to smoke after the new information became available, might be more myopic than quitters and persons who did not begin to smoke. One explanation for the much stronger negative relation between smoking and education in the 1970s and 1980s than prior to the Surgeon General's report is that more educated people tend to have lower rates of preference for the present. Presumably, this is partly why they accept the delayed benefits of higher education. Farrell and Fuchs (1982) do show that the negative association between education and smoking is not fully explained by any effects of education on the propensity to smoke.

The behavior of teenagers is persuasive evidence of forwardlooking behavior by smokers. Teenagers are often said to be among the most impatient (see the questionnaire evidence in Davids and Falkoff [1975]). If so, their propensity to smoke should be hardly affected by health consequences -delayed for 20 or more years, although parental disapproval may have a big effect. Yet smoking rates of males between ages 21 and 24 declined by over one-third from 1964 to 1975 (see Harris 1980).

The long-run change in the consumption of addictive goods due to a change in wealth also exceeds the short-run change because the stock of consumption capital would change over time until a new steady state is reached (Spinnewyn [ 1981, p. 101 ] has a similar result for wealth effects). By differentiating the first-order conditions in equation (6) with respect to μ, the marginal utility of wealth, we get the response of steady-state consumption to a change in wealth (if the utility function is quadratic):

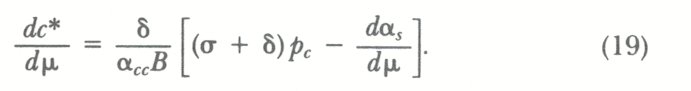
Since μ and wealth are negatively related, c is a superior or inferior good as dc*/dμ ≤ 0. If wealth rises because of an increase in earnings, the term dα3 /dμ is likely to be positive for harmfully addictive goods because the negative effect on earnings of increased consumption is likely to be greater when earnings are greater (dμw3/dμ > 0). For example, heavy drinking on the job reduces the productivity of an airline pilot or doctor more than that of a janitor or busboy. Equation (19) shows that c would be an inferior good if the negative effect on earnings were sufficiently large. Therefore, the spread of information about the health hazards of smoking should have reduced the income elasticity of smoking, and it could have made smoking an inferior good. This elasticity apparently did decline after the 1960s to a negligible level (see Schneider, Klein, and Murphy 1981). Since women earn less than men, this may help explain why smoking by women has grown relative to smoking by men during the past 25 years.

VI. Temporary Changes in Price and Life Cycle Events

If utility and earnings functions are quadratic, the demand for c at each moment in time can be explicitly related to the initial S and to past and future prices of c (see eq. [A2] in App. A). Both past and future prices affect current consumption, but the effects are not symmetrical. Changes in past prices affect current consumption by changing the current stock of consumption capital, whereas changes in future prices affect current consumption by changing current full prices through the effects on future stocks and future consumption.

The effect on consumption of a differential change in price over a small interval divided by the length of this interval has a nonzero limit as the length of the interval goes to zero. Equation (A2) implies that these limits are

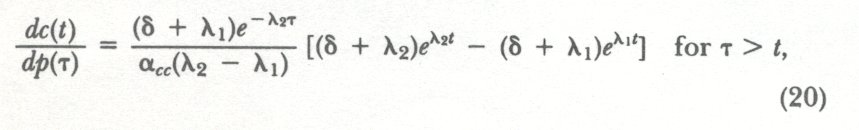
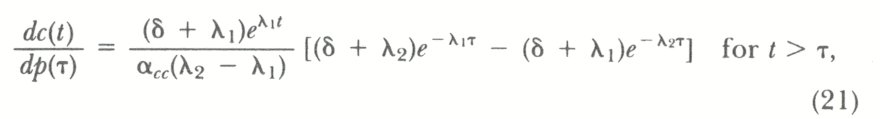
where changes in price are compensated to hold the marginal utility of wealth constant, and a 2 and k, are the larger and smaller roots of equation (12).

The important implication of these equations is that the signs of both cross-price derivatives depend only on the sign of δ + λ¹. Section III shows that this term is positive with adjacent complementarity and negative with adjacent substitution. Since the terms in brackets are always positive and αcc is negative, dc(t)/dp(T) will be negative if and only if δ + λ¹ is positive. Hence, adjacent complementarity is a necessary and sufficient condition for negative compensated cross-price effects.

A negative cross derivative when marginal utility of income is held constant is a common definition of complementarity in consumption theory. Therefore, adjacent complementarity is a necessary and sufficient condition for present and future consumption, and for present and past consumption, to be complements. This conclusion strongly qualifies the claim by Ryder and Heal that adjacent "complementarity ... is different from complementarity in the Slutsky sense" (1973, p. 4). Since our definition of potential addiction is linked to adjacent complementarity, a good is addictive if and only if consumption of the good at different moments in time are complements. Moreover, the degree of addiction is stronger when the complementarity in consumption is greater.

The link between addiction and complementarity implies that an anticipated increase in future prices of addictive goods lowers current consumption. These negative effects of anticipated future price changes on the present consumption of addictive goods are a major way to distinguish rational addiction or rational habit formation from myopic behavior (myopic behavior is assumed, e.g., by Pollak [1970, 1976], von Weizsacker [ 1971 ], and Phlips [ 1974]).

The longer that future price changes are anticipated, the bigger is their effect on the current consumption of addictive goods. In equation (20), where T > t, an increase in T (with T - t held constant) increases the absolute value of dc(t)/dp(T) if λ¹ + δ > 0. The reason is that the longer a future price rise of an addictive good is anticipated, the greater is the reduction in past consumption of the good. Therefore, the smaller would be the stock of capital carried into the present period. We are not merely restating the familiar result that elasticities of demand are greater when price changes are anticipated since the elasticity for goods with adjacent substitution (λ¹ + δ < 0) is smaller when future price changes have been anticipated for a longer period of time.

Equation (21) shows that recent past prices have larger effects on current consumption than more distant past prices when steady states are stable. However, with an unstable steady state, changes in consumption at one point in time lead to larger and larger changes in future consumption since consumption capital continues to grow.

The permanent changes in stationary price considered in Section V can be said to combine changes in price during the present period with equal changes in price during all future periods. Since a (compensated) future price increase of an addictive good reduces its current consumption, an increase only in its current price has a smaller effect on current consumption than a permanent increase in its price.

The complementarity between present and future consumption is larger for more addictive goods. Therefore, permanent changes in prices of addictive goods might have large effects on their current consumption. Although our analysis implies that rational addicts respond more to price in the long run than in the short run, they may also respond a lot in the short run.

The beginning and resumption of harmful addictions, such as smoking, heavy drinking, gambling, cocaine use, and overeating, and of beneficial addictions, such as religiosity and jogging, are often traceable to the anxiety, tension, and insecurity produced by adolescence, marital breakup, job loss, and other events (see the many studies reviewed in Peele [ 1985, chap. 5]). This suggests that consumption of many harmfully addictive goods is stimulated by divorce, unemployment, death of a loved one, and other stressful events. If these events lower utility while raising the marginal utility of addictive goods, then changes in life cycle events have the same effect on consumption as changes in prices (see App. A). For example, a compensated increase in stress during a future finite time interval raises future c's and future S's. The same reasoning used to show that declines in future prices raise present consumption of addictive goods shows that anticipated future stress raises the current consumption of addictive goods if it raises future consumption.

Therefore, even persons with the same utility function and the same wealth who face the same prices may have different degrees of addiction if they have different experiences. However, to avoid the unattractive implication of equation (2) that all persons who never consumed an addictive good-such as teenagers who never smoked-would have a zero initial stock of consumption capital, we assume that some events directly affect the stock of consumption capital. If Z(t) is the rate of such events at time t, the stock adjustment equation would be changed to

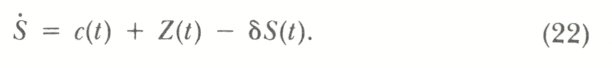
Even if c had not been consumed in the past, S would vary across individuals because of different experiences (Z). Appendix A analyzes the effects of past and future Z's on the current consumption of c.

Temporary events can permanently "hook" rational persons to addictive goods. For example, a person may become permanently addicted to heroin or liquor as a result of peer pressure while a teenager or of extraordinary stress while fighting in Vietnam. If adolescence or a temporary assignment in Vietnam raises demand for c in figure 2 from c¹ to c², he would temporarily move along the path p²p² from c¹2 to c2. At that point-when the stress ceases-he abruptly returns to the path p¹p¹ at cí (Note that his consumption during the stressful events is affected by how temporary they are.) In this example, he accumulates sufficient capital while under stress to remain hooked afterward. Starting at c I, he would have eventually abstained if he had never been subject to such stress, but instead he ends up with a sizable steady-state consumption. Although most Vietnam veterans did end their addiction to drugs after returning to the United States, many did not, and others shifted from dependence on drugs to dependence on alcohol (see Robins et al. 1980).

Some critics claim that the model in Stigler and Becker (1977)presumably also the model in this paper-is unsatisfactory because it implies that addicts are "happy," whereas real-life addicts are often discontented and depressed (see, e.g., Winston 1980). Although our model does assume that addicts are rational and maximize utility, they would not be happy if their addiction results from anxiety-raising events, such as a death or divorce, that lower their utility. Therefore, our model recognizes that people often become addicted precisely because they are unhappy. However, they would be even more unhappy if they were prevented from consuming the addictive goods.

It might seem that only language distinguishes our approach to the effects of events on addictions from approaches based on changes in preferences. But more than language is involved. In many of these other approaches, different preferences or personalities fight for control over behavior (see Yaari 1977; Elster 1979; Winston 1980; Schelling 1984). For example, the nonaddictive personality makes commitments when in control of behavior that try to reduce the power of the addictive personality when it is in control. The nonaddictive personality might join Alcoholics Anonymous, enroll in a course to end smoking, and so forth (see the many examples in Schelling [1984]). By contrast in our model, present and future consumption of addictive goods are complements, and a persor becomes more addicted at present when he expects events to raise his future consumption. That is, in our model, both present and future behavior are part of a consistent maximizing plan.

VII.   Cold Turkey and Binges

Our theory of rational addiction can explain why many severe addictions are stopped only with "cold turkey," that is, with abrupt cessation of consumption. Indeed, it implies that strong addictions end only with cold turkey. A rational person decides to end his addiction if events lower either his demand for the addictive good sufficiently or his stock of consumption capital sufficiently. His consumption declines over time more rapidly when a change in current consumption has a larger effect on future consumption. The effect on future consumption is larger when the degree of complementarity and the degree of addiction are stronger. Therefore, rational persons end stronger addictions more rapidly than weaker ones.

If the degree of complementarity and potential addiction became sufficiently strong, the utility function in equation (1) would no longer be concave. Appendix B shows that the relation between present consumption of an addictive good (c) and its past consumption (S) can then become discontinuous at a point (Ŝ) (see fig. 2), such that c > δŜ when S > Ŝ and c < δŜ when S < Ŝ. Although Ŝ is not a steady-state stock, it plays a role similar to that of an unstable steady-state stock when the utility function is concave. If S is even slightly less than Ŝ, consumption falls along p³ to zero over time. Similarly, if S is even slightly above Ŝ, consumption rises over time along p³, perhaps to a stable level. However, a decline in S from just above to just below Ŝ causes an infinite rate of fall in c because the relation between c and S is discontinuous at Ŝ. Indeed, with a sufficiently large discontinuity, an addict who quits would use cold turkey; that is, he would immediately stop consuming once he decides to stop.

The explanation of this discontinuity is straightforward. If S is even slightly bigger than Ŝ, the optimal consumption plan calls for high c in the future because the good is highly addictive. Strong complementarity between present and future consumption then requires a high level of current c. If S is even slightly below Ŝ, future c will be very low because the addiction ends quickly, and strong complementarity then requires a low level of current c.

Clearly, then, quitting by cold turkey is not inconsistent with our theory of rational addiction. Indeed, our theory even requires strong addictions to terminate with cold turkey. Moreover, when complementarity is sufficiently strong to result in a nonconcave utility function, we generate sharp swings in consumption in response to small changes in the environment when individuals either are beginning or are terminating their addiction.

The short-run loss in utility from stopping consumption gets bigger as an addiction gets stronger. Yet we have shown that rational persons use cold turkey to end a strong addiction even though the short-run "pain" is considerable. Their behavior is rational because they exchange a large short-term loss in utility for an even larger long-term gain. Weak wills and limited self-control are not needed to understand why addictions to smoking, heroin, and liquor can end only when the consumption stops abruptly.

A rational addict might postpone terminating his addiction as he looks for ways to reduce the sizable short-run loss in utility from stopping abruptly. He may first try to stop smoking by attending a smoking clinic but may conclude that this is not a good way for him. He may try to substitute gum chewing and jogging for smoking. These too may fail. Eventually, he may hit on a successful method that reduces the short-term loss from stopping. Nothing about rationality rules out such experiments and failures. Indeed, rationality implies that failures will be common with uncertainty about the method best suited to each person and with a substantial short-run loss in utility from stopping.

The claims of some heavy drinkers and smokers that they want to but cannot end their addictions seem to us no different from the claims of single persons that they want to but are unable to marry or from the claims of disorganized persons that they want to become better organized. What these claims mean is that a person will make certain changes-for example, marry or stop smoking-when he finds a way to raise long-term benefits sufficiently above the shortterm costs of adjustment.

"Binges" are common in alcoholism, overeating, and certain other addictions. We define a binge as a cycle over time in the consumption of a good. Binging may seem to be the prototype of irrational behavior, yet a small extension of our model makes binging consistent with rationality.

Consider overeating. Weight rises and health falls as eating increases. We assume that two stocks of consumption capital determine current eating: call one stock weight and the other "eating capital." Our analysis so far, in effect, has absorbed weight and eating capital into a single stock (S). We readily generate binges if the two stocks have different depreciation rates and different degrees of complementarity and substitutability with consumption.

To get cycles of overeating and dieting, one stock (say eating capital) must be complementary with eating and have the higher depreciation rate, while the other stock (weight) must be substitutable (see eq. [C8] in App. C). Assume that a person with low weight and eating capital became addicted to eating. As eating rose over time, eating capital would rise more rapidly than weight because it has the higher depreciation rate.

Ultimately, eating would level off and begin to fall because weight continues to increase. Lower food consumption then depreciates the stock of eating capital relative to weight, and the reduced level of eating capital keeps eating down even after weight begins to fall. Eating picks up again only when weight reaches a sufficiently low level. The increase in eating then raises eating capital, and the cycle begins again. These cycles can be either damped or explosive (or constant) depending on whether the steady state is stable or unstable.

Although, as is usual in such problems, two capital stocks are needed to get cycles (Ryder and Heal [1973] also get cycles in consumption with two capital stocks), these stocks in our analysis have a plausible interpretation in terms of differences in rates of depreciation and degrees of complementarity and substitutability. In our analysis, binges do not reflect inconsistent behavior that results from the struggle among different personalities for control. Rather, they are the outcome of consistent maximization over time that recognizes the effects of increased current eating on both future weight and the desire to eat more in the future.

VIII. Summary and Conclusions

In our theory of rational addiction, "rational" means that individuals maximize utility consistently over time, and a good is potentially addictive if increases in past consumption raise current consumption. We show that steady-state consumption of addictive goods is unstable when the degree of addiction is strong, that is, when the complementarity between past and current consumption is strong. Unstable steady states are a major tool in our analysis of addictive behavior. Consumption rises over time when above unstable steady-state levels, and it falls over time, perhaps until abstention, when below unstable steady states.

Addictions require interaction between a person and a good. Obviously, cigarettes and heroin are more addictive than sweaters and sherbet. Yet not all smokers and heroin users become addicted. We show that, other things the same, individuals who discount the future heavily are more likely to become addicted. The level of incomes, temporary stressful events that stimulate the demand for addictive goods, and the level and path of prices also affect the likelihood of becoming addicted.

Permanent changes in prices of addictive goods may have a modest short-run effect on the consumption of addictive goods. This could be the source of a general perception that addicts do not respond much to changes in price. However, we show that the long-run demand for addictive goods tends to be more elastic than the demand for nonaddictive goods.

Anticipated future increases in price reduce current consumption of addictive goods because their consumption at different moments of time are complements. This implies that temporary changes in the price of an addictive good have smaller effects on current consumption than (compensated) permanent changes.

Strong addictions to smoking, drinking, and drug use are usually broken only by going cold turkey, that is, by abrupt withdrawal. The need for cold turkey may suggest a weak will or other forms of lessthan-rational behavior. Yet we show that cold turkey is consistent with rational behavior. Indeed, rational persons end strong addictions only with rapid and sometimes discontinuous reductions in consumption.

Addiction is a major challenge to the theory of rational behavior. Not only cigarettes, alcoholic beverages, and cocaine are obviously addictive, but many other goods and activities have addictive aspects. We do not claim that all the idiosyncratic behavior associated with particular kinds of addictions are consistent with rationality. However, a theory of rational addiction does explain well-known features of addictions and appears to have a richer set of additional implications about addictive behavior than other approaches. This is the challenge posed by our model of rational addiction.

Appendix A

If the utility function is quadratic, if the events Z(t) affect the stock of consumption capital (see eq. [22]), and if the events E(t) affect the utility function, then the capital stock is a solution to the differential equation

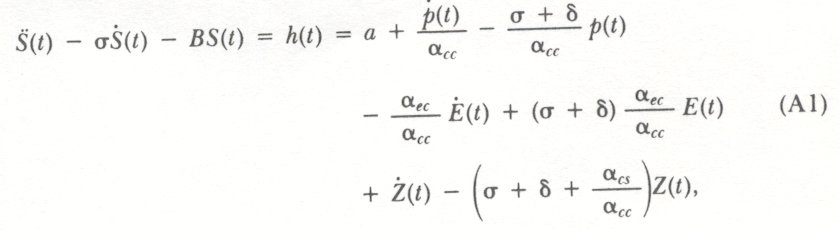
where μp(t) has been replaced by p(t) to save on notation, αec is the coefficient of E(t)c(t), a = (δ + σ)(α/αcc) + ((α/αcc)¹ and B is given by equation (11). With the relation between S and c in equation (22), the solution to this equation for c(t) that satisfies the initial condition S(0) = S° and the transversality condition in equation (8) is

Therefore, future events that raise the stock have a negative effect on current consumption independent of whether there is adjacent complementarity.

Appendix B

If the degree of adjacent complementarity is sufficiently strong that the utility function is no longer concave in c(t) and S(t), the two roots in equation (12) will be complex, and the form of the optimal consumption path will change significantly. We consider the case in which the utility function is still concave in c(t) and S(t) separately, but it is not jointly concave in c(t) and S(t):

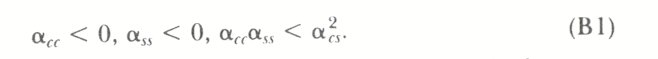
These assumptions indicate that a high degree of complementarity between past and current consumption-that is, between c(t) and S(t)-is what creates some convexity in the utility function, not a lack of concavity in either c(t) or S(t) alone. In regions in which 4B < -σ², both roots of the characteristic equation λ² - σλ - B will be complex (see eq. [13]).

If the roots are complex, the unstable steady state is replaced by a discontinuity in the optimal consumption function that relates consumption c to the current stock S. However, as long as the utility function satisfies αcc < 0, then this discontinuity will always be of a particular form: c(S) δŜ to the right of Ŝ.

If Ŝ is above a lower steady state at abstinence, the critical stock can generate the phenomenon of quitting cold turkey. That is, consumption could fall considerably in response to even a small rise in price or a "small" event.

A simple example may be the best way to illustrate this result. Unfortunately, quadratic utility functions that satisfy the inequalities in equation (B 1) have unbounded utility if the horizon is infinite. However, consider the following modified quadratic utility function:

c(t) = δS* are all solutions to the Euler equation. However, the convexity induced by the strong complementarity between c and S implies that lifetime utility will be maximized by choosing either c(t) = 0 or c(t) = C when the initial stock is S*.

Appendix C

We assume a quadratic utility function in c, y, and two stocks, S¹ and S², where S¹ and S² do not interact (α¹² = 0). While the steady-state results are similar for the two-stock and one-stock models, the dynamics are quite different. To simplify notation, we transform the definitions of the stocks and consumption so that the steady-state values of c and S are zero. The solution to this standard control problem is of the form

JJ Equations (C7) and (C8) together show that complex roots require the stock with the higher depreciation rate to have adjacent complementarity and the other stock to have adjacent substitution.

References

Becker, Gary S.; Grossman, Michael; and Murphy, Kevin M. "An Empirical Analysis of Cigarette Addiction." Paper presented at the Workshop in Applications of Economics, Univ. Chicago, May 18, 1987.

Becker, Gary S., and Murphy, Kevin M. "A Theory of Rational Addiction." Working Paper no. 41. Chicago: Univ. Chicago, Center Study Econ. and State, September 1986.

Boyer, Marcel. "A Habit Forming Optimal Growth Model." Internal. Econ. Rev. 19 (October 1978): 585-609.

Boyer, Marcel. "Rational Demand and Expenditures Patterns under Habit Formation." J. Econ. Theory 31 (October 1983): 27-53.

Cook, Philip J., and Tauchen, George. "The Effect of Liquor Taxes on Heavy Drinking." Bell J. Econ. 13 (Autumn 1982): 379-90.

Davids, Anthony, and Falkoff, Bradley B. "Juvenile Delinquents Then and Now: Comparisons of Findings from 1959 and 1974."J. Abnormal Psychology 84 (April 1975): 161-64.

Donegan, Nelson H.; Rodin, Judith; O'Brien, Charles P.; and Solomon, Richard L. "A Learning-Theory Approach to Commonalities." In Com monalities in Substance Abuse and Habitual Behavior, edited by Peter K. Levison, Dean R. Gerstein, and Deborah R. Maloff. Lexington, Mass.: Heath, 1983.

Elster, Jon. Ulysses and the Sirens: Studies in Rationality and Irrationality. Cambridge: Cambridge Univ. Press, 1979.

Farrell, Phillip, and Fuchs, Victor R. "Schooling and Health: The Cigarette Connection." J. Health Econ. 1 (December 1982): 217-30.

Harris, Jeffrey E. "Cigarette Smoking among Women and Men in the United States, 1900-1979." In 1980 Surgeon General's Report on Smoking and Health: The Health Consequences of Smoking for Women. Washington: U.S. Department of Health, Education, and Welfare, 1980.

Iannaccone, Laurence R. "Consumption Capital and Habit Formation with an Application to Religious Participation." Ph.D. dissertation, Univ. Chicago, 1984.

Iannaccone, Laurence R Addiction and Satiation." Econ. Letters 21, no. 1 (1986): 95-99.

Ippolito, Richard A.; Murphy, R. Dennis; and Sant, Donald. Staff Report on Consumer Responses to Cigarette Health Information. Washington: Fed. Trade Comm., 1979.

Mullahy, John. "Cigarette Smoking: Habits, Health Concerns, and Heterogeneous Unobservables in a Macro-economic Analysis of Consumer Demand." Ph.D. dissertation, Univ. Virginia, 1985.

Peele, Stanton. The Meaning of Addiction: Compulsive Experience and Its Interpre tation. Lexington, Mass.: Lexington, 1985.

Phlips, Louis. Applied Consumption Analysis. Amsterdam: North-Holland, 1974.

Pollak, Robert A. "Habit Formation and Dynamic Demand Functions."J.P.E. 78, no. 4, pt. 1 (July/August 1970): 745-63.

Pollak, Robert A."Habit Formation and Long-Run Utility Functions."J. Econ. Theory 13 (October 1976): 272-97.

Robins, Lee N.; Helzer, John E.; Hesselbrook, Michi; and Wish, Eric. "Vietnam Veterans Three Years after Vietnam: How Our Study Changed Our View of Heroin." In The Yearbook of Substance Use and Abuse, vol. 2, edited by Iron Brill and Charles Winick. New York: Human Sciences, 1980.

Ryder, Harl E., Jr., and Heal, Geoffrey M. "Optimum Growth with Intertemporally Dependent Preferences." Rev. Econ. Studies 40 (January 1973): 1 33.

Schelling, Thomas C. Choice and Consequence. Cambridge, Mass.: Harvard Univ. Press, 1984.

Schneider, Lynne; Klein, Benjamin; and Murphy, Kevin M. "Governmental Regulation of Cigarette Health Information."J. Law and Econ. 24 (December 1981): 575-612.

Spinnewyn, Frans. "Rational Habit Formation." European Econ. Rev. 15 (January 1981): 91-109.

Stigler, George J., and Becker, Gary S. "De Gustibus Non Est Disputandum." A.E.R. 67 (March 1977): 76-90.

von WeizsAcker, Carl Christian. "Notes on Endogenous Change of Tastes."J. Econ. Theory 3 (December 1971): 345-72.

Winston, Gordon C. "Addiction and Backsliding: A Theory of Compulsive Consumption." J. Econ. Behavior and Organization 1 (December 1980): 295 324.

Yaari, Menahem E. "Consistent Utilization of an Exhaustible Resource, or How to Eat an Appetite-arousing Cake." Working paper. Jerusalem: Hebrew Univ., Center Res. Math. Econ. and Game Theory, 1977.